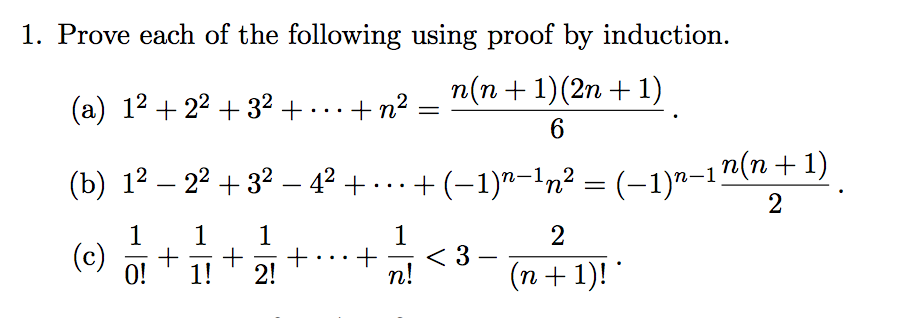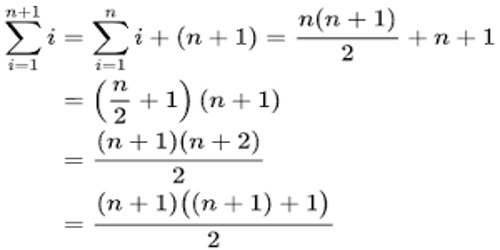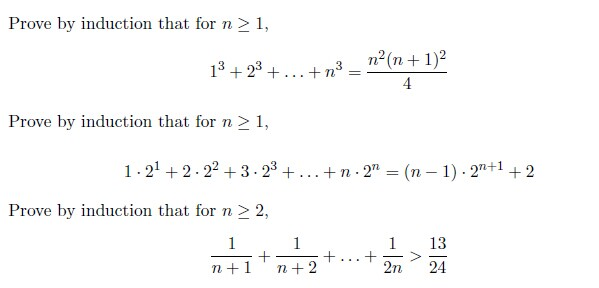N N 1 2 Proof By Induction

In this case 2.

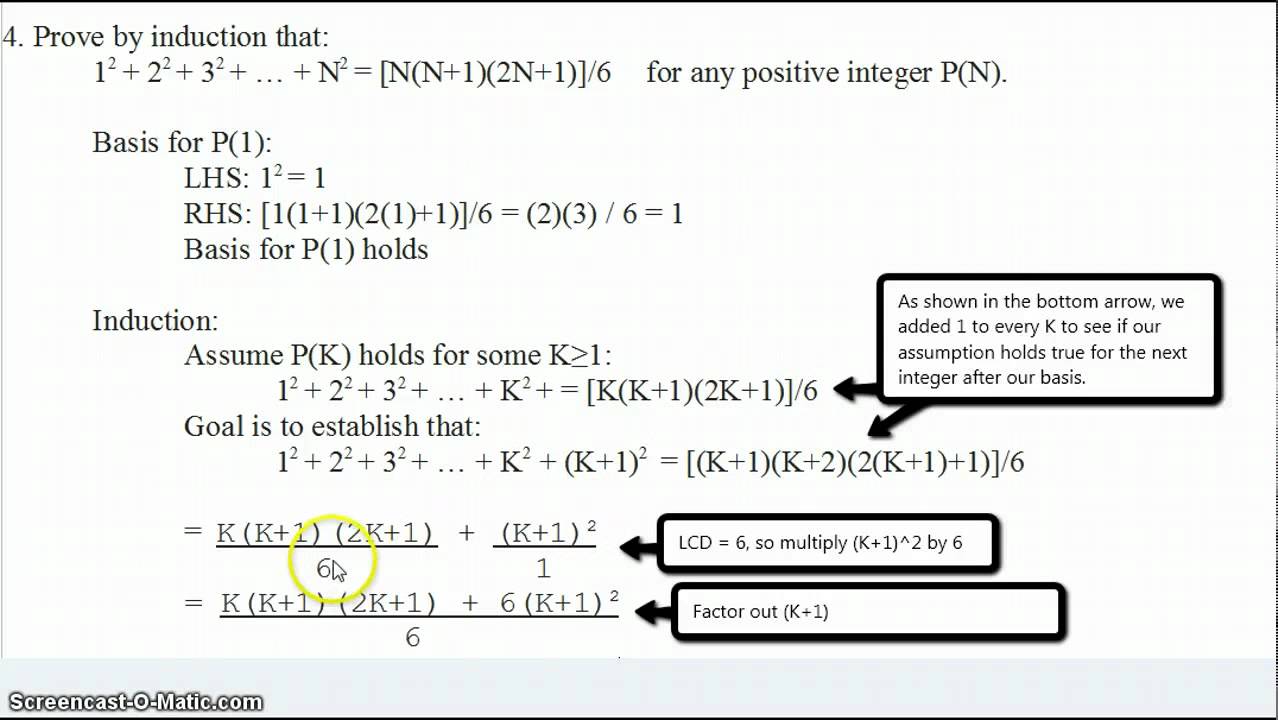
N n 1 2 proof by induction. Induction proofs have four components. N 1 means the first value of the expression on the left side. Compute answers using wolfram s breakthrough technology knowledgebase relied on by millions of students professionals. There is a pitfall to avoid here.

Show that given any positive integer n n 3 2 n yields an answer divisible by 3. An example showing how to do a proof by mathematical induction show that for all n 2 4 6. Did not show it is true for any values. Show the equation is true for n 1 n 2.

2n n n 1 step 1. Prove by induction sum of j from 1 to n n n 1 2 for n 0. N and k are just variables. Inequality proofs seem particularly difficult when they involve powers of n but they can be managed just like any other i.

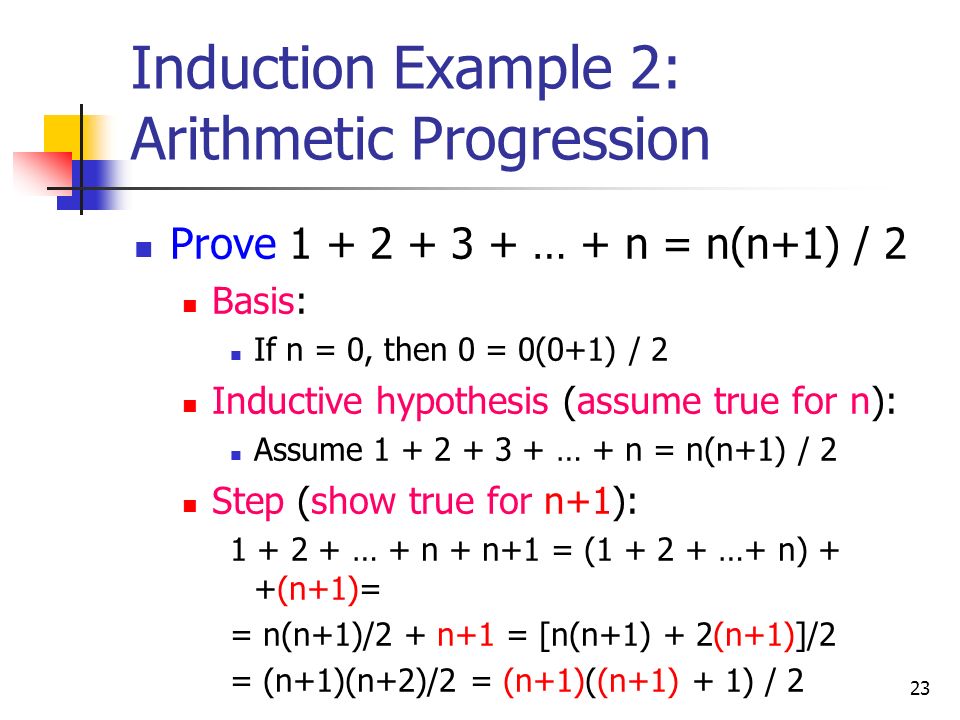
The given statement let p n. Is the set of integers for n infinite. Now what i want to do in this video is prove to you that i can write this as a function of n that the sum of all positive integers up to and including n is equal to n times n plus one all of that over 2. Bernard s answer highlights the key algebraic step but i thought i might mention something that i have found useful when dealing with induction problems.

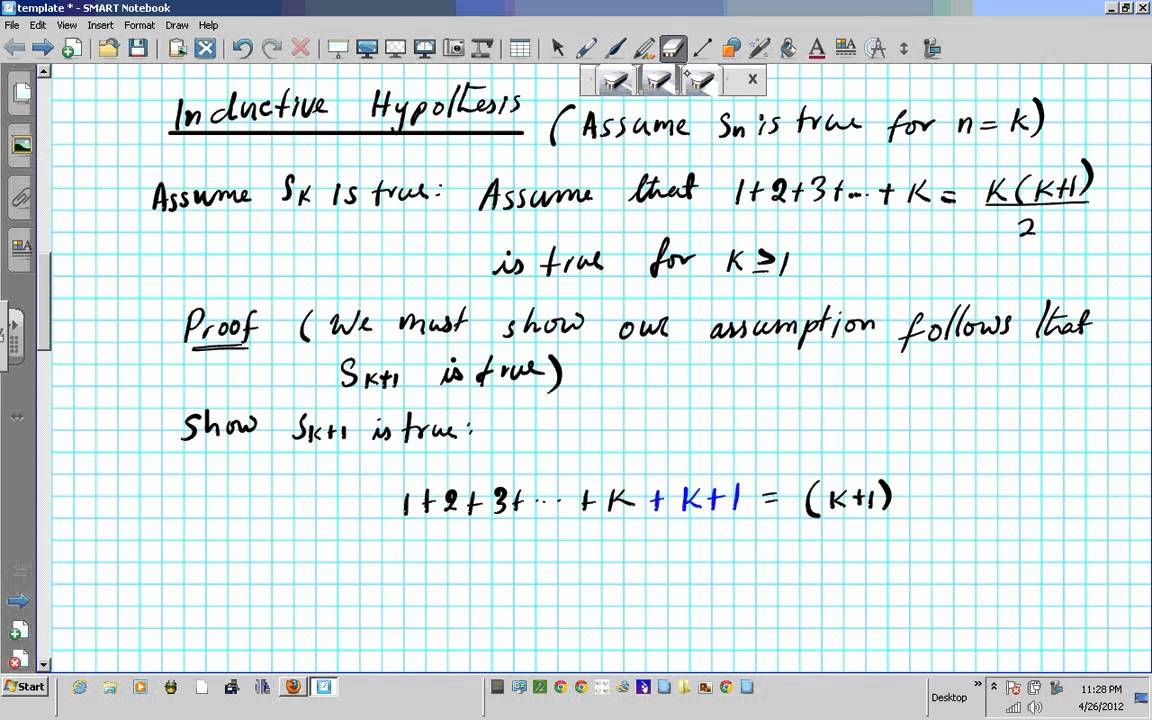
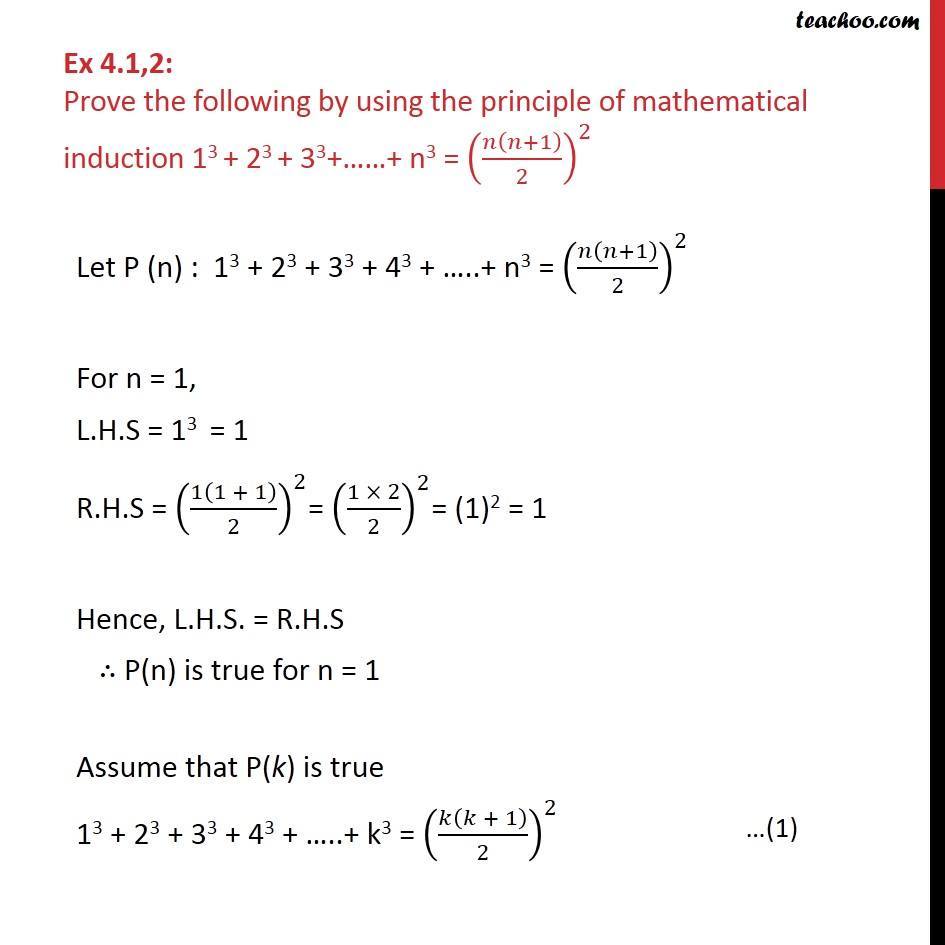
Go through the first two of your three steps. Proof by induction prove the formula works for all cases. N 3 2 n is divisible by 3. The assumption step assume true for n k 4.

N n n 1 2 for n n is a natural number step 1. And the way i m going to prove it to you is by induction. The induction step now let n k 1. 1 2 3.

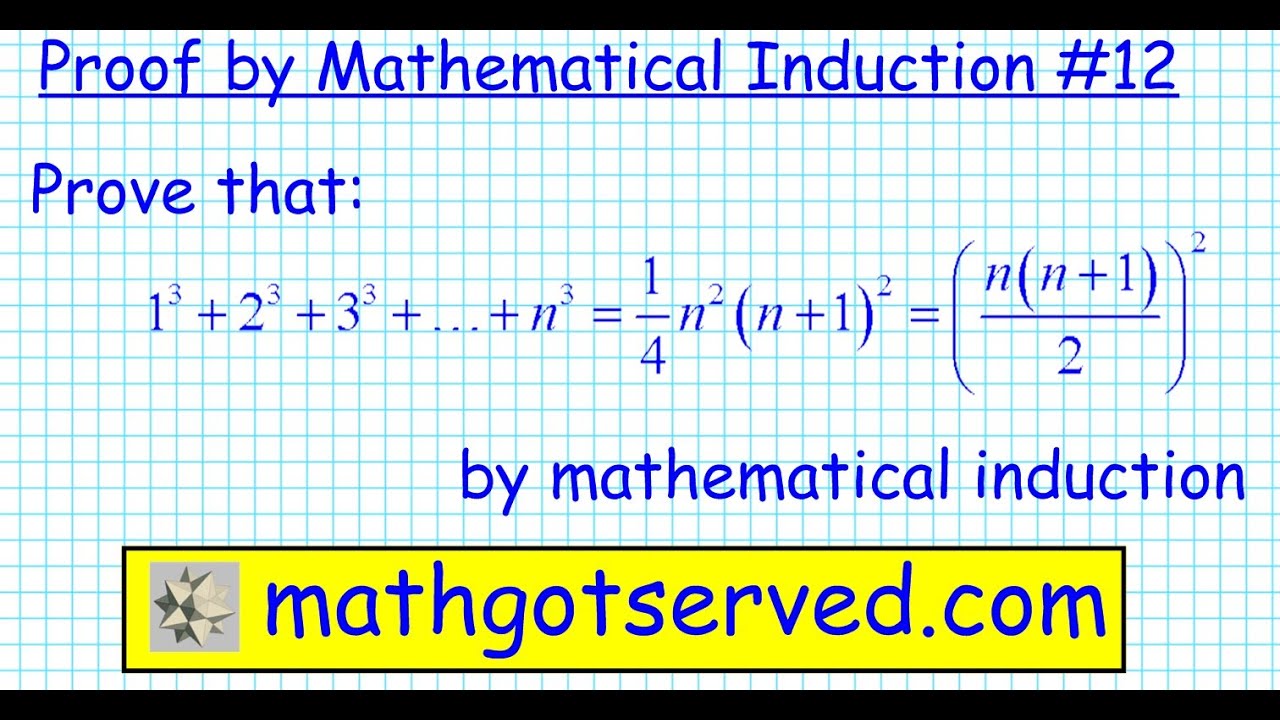
Whenever you have an induction problem like this that involves a sum rewrite the sum using sigma notation. Prove 1 2 3. N n n 1 2 step. Here is a more reasonable use of mathematical induction.

For math science nutrition history geography engineering. So our property p is.